
Calc bc review series#
In fact, this series converges to the value 2. This series is the sum of the reciprocals of the powers of 2. On the other hand, the following series converges: In this case, the general term a n = n itself blows up to ∞. In fact, any series whose general terms a n do not tend to zero will diverge. More precisely, the partial sums are unbounded. That makes sense, right? If you keep adding larger numbers, the running total just gets bigger and bigger. The series of all natural numbers (counting numbers) clearly diverges to infinity. Here are some easy examples to get you started. Next would be s 5 having five terms, and so on.īy definition, the series Σ a n converges to a sum S if and only if the sequence of partial sums converges to S. Of course, the list of partial sums goes on forever. So for example, the first four partial sums of a series are: The kth partial sum for a series Σ a n is the sum of the first k terms of the series: The precise definition for convergence of a series has to do with its partial sums. Here, we would say the series diverges (but not to ∞ nor -∞). As you add term after term, the value of the sum keeps jumping around or oscillating among multiple values. In that case, we say that the series diverges to negative infinity (-∞)

Then we say that the series diverges to infinity (∞). The sum gets larger and larger without bound as you add more terms.Otherwise, the series diverges.Ī series may diverge in three different ways: If the series has a finite value, then we say it converges to that value. Often, a n will be expressed as a formula in terms of n. We say that a n is the general term for the series.

And both notations stand for the same thing. You may see the sum written out term by term or in sigma notation.
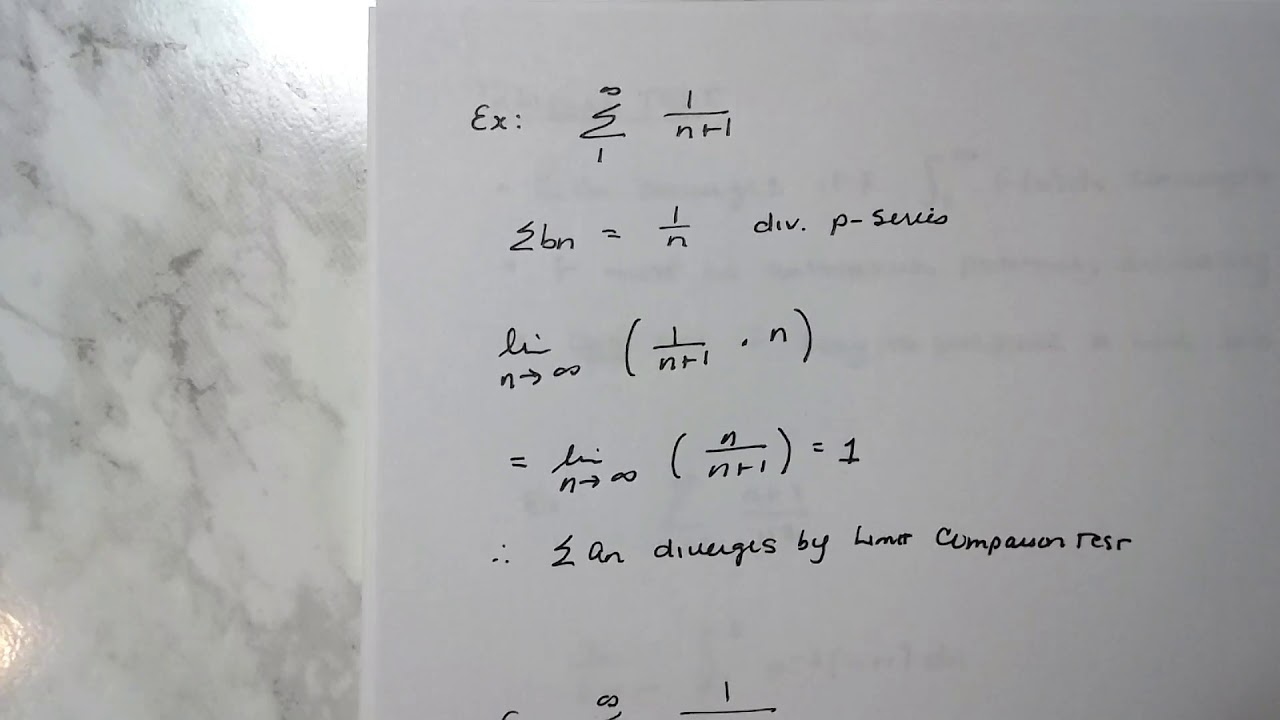
Nothing more, nothing less! For all the essential details about sequences, you can check out: AP Calculus BC Review: Sequences and Convergence.Ī series, on the other hand, is the sum of a sequence. Series and Sequencesįirst of all, the two terms, sequence and series, are not interchangeable.Ī sequence is a list of numbers. Once you have the series fundamentals down, then you’ll be able to tackle more advanced topics concerning series! Series Fundamentals Then we’ll take a look at a few example problems from the AP Calculus BC exam. In this article, we’ll review the definition of a series. Good question! Read on to catch up on your series fundamentals!


 0 kommentar(er)
0 kommentar(er)
